WHAT IS SIESTA?
SIESTA (Spanish Initiative for Electronic Simulations with Thousands of Atoms) is both a method and its computer program implementation (Fortran 95), to perform efficient electronic structure calculations and ab initio molecular dynamics simulations of molecules and solids.
- SIESTA has excellent performance for finite systems such as slabs, surfaces, clusters, nanotubes and molecules.
- SIESTA can easily tackle computationally demanding systems (>10000 atoms), which are out of the reach of plane-wave codes.
- SIESTA has efficient parallelisation implemented over orbitals or over k-points.
SOLVERS
- Energy optimisation (various algorithms for coordinates and cell relaxation)
- Ab initio Molecular Dynamics
- Ballistic electron transport under non-equilibrium (TranSIESTA)
- Linear Scaling regime
FEATURES
- Standard Kohn-Sham self-consistent density functional theory (DFT) in the local density (LDA-LSD), generalized gradient (GGA) approximations, hybrid functionals (HSE) and non-local van der Waals interactions (VDW-DF).
- Norm-conserving pseudopotentials in nonlocal (Kleinman-Bylander) form.
- Use of atomic orbitals as a basis set, allowing unlimited multiple-zeta and angular momenta, polarization and off-site orbitals.
- Hartree and exchange-correlation potentials are calculated using real-space projection of wavefunctions and density.
- k-points sampling of the Brillouin zone.
- Spin polarised calculations.
- Non-collinear spin calculations of magnetic systems.
- On-site Coulomb corrections DFT+U.
- Spin-orbit coupling (SOC).
- Time dependent density functional theory (TDDFT).
WHAT SIESTA CAN COMPUTE?
By design SIESTA is very efficient for the description of finite systems as surfaces, adsorbates, nanotubes, nano-clusters, biological molecules, amorphous semiconductors, ferroelectric films, low-dimensional metals. This is one of the main advantage of SIESTA over plane-wave codes.
- Total and partial energies
- Atomic forces
- Stress tensor
- Electric dipole moment
- Macroscopic Polarisation
- Born Effective Charges
- Atomic, orbital and bond populations
- Electron density
- Charge analysis
- Magnetic moment
- Optical properties (imaginary part of the dielectric function)
- Local and orbital-projected density of states
- Band structure
- COOP and COHP curves for chemical bonding analysis
- Phonons
EFFICIENT PARALLELISATION
LINEAR SCALING
SIESTA allows the use of localised linear combinations of the occupied orbitals (valence-bond or Wannier-like functions), making the computer time and memory scale linearly with the number of atoms. Linear scaling algorithm enables simulations of tens of thousands atoms (>104) feasible using a modest workstation. Linear scaling O(N) works for systems with a finite HOMO-LUMO separation.
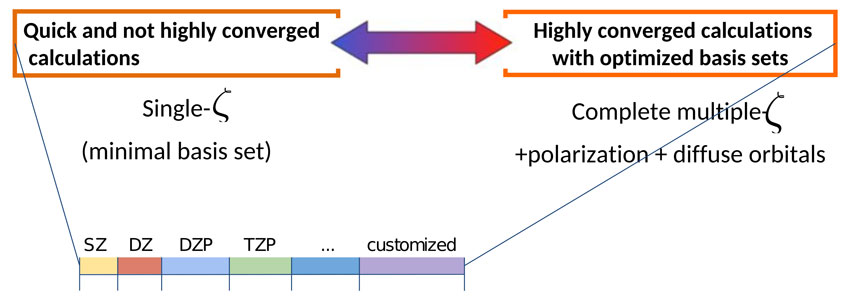
BASIS SET
SIESTA’s efficiency stems from the use of strictly localized basis sets and from the implementation of linear-scaling algorithms, which can be applied to suitable systems. The radial shape of every orbital is numerical, and any shape can be used and provided by the user, with the only condition that it has to be strictly zero beyond a distance from the corresponding nucleus. Finite-support basis sets are the key for calculating the Hamiltonian and overlap matrices in O(N) operations.
TRANSIESTA
SIESTA includes the TranSIESTA solver, which provides the ability to model open-boundary systems, where ballistic electron transport is taking place. TranSIESTA makes possible computing electronic transport properties, such as the zero-bias conductance and the I-V characteristic, of a nanoscale system in contact with two electrodes at different electrochemical potentials.
DISTRIBUTION
SIESTA is an open source code. Since the 13th of May 2016, with the 4.0 version announcement, SIESTA was released under the terms of the GPL open-source license. Source packages and access to the development versions can be obtained from the new development and distribution platform.
In 2015, SIMUNE established a strategic partnership with SIESTA offering support, training, and future development of the SIESTA code to facilitate materials design challenges. SIMUNE develops the project: SIESTA-PRO – Spanish Initiative for Electronic Simulations with Thousands of Atoms: Open Source code with professional support and warranty.
HOW TO CITE SIESTA:
- Artacho, E. , Sánchez‐Portal, D. , Ordejón, P. , García, A. and Soler, J. M. (1999), Linear‐Scaling ab‐initio Calculations for Large and Complex Systems. phys. stat. sol. (b), 215: 809-817. doi:10.1002/(SICI)1521-3951(199909)215:1<809::AID-PSSB809>3.0.CO;2-0
- Emilio Artacho, E. Anglada, O. Diéguez, J. D. Gale, A. García, J. Junquera, R. M. Martin, P. Ordejón, J. M. Pruneda, D. Sánchez-Portal and J. M. Soler, The SIESTA method; developments and applicability. Journal of Physics: Condensed Matter, 20 (6), 064208, (2008), url: https://stacks.iop.org/0953-8984/20/i=6/a=064208
- José M Soler, Emilio Artacho, Julian D Gale, Alberto García, Javier Junquera, Pablo Ordejón and Daniel Sánchez-Portal, Journal of Physics: Condensed Matter, 14 (11), (2002), url: https://iopscience.iop.org/article/10.1088/0953-8984/14/11/302
Do you have a question?
Contact us. Simune Team would be happy to hear from you.
Would like to try ASAP trial?
Make a request.




